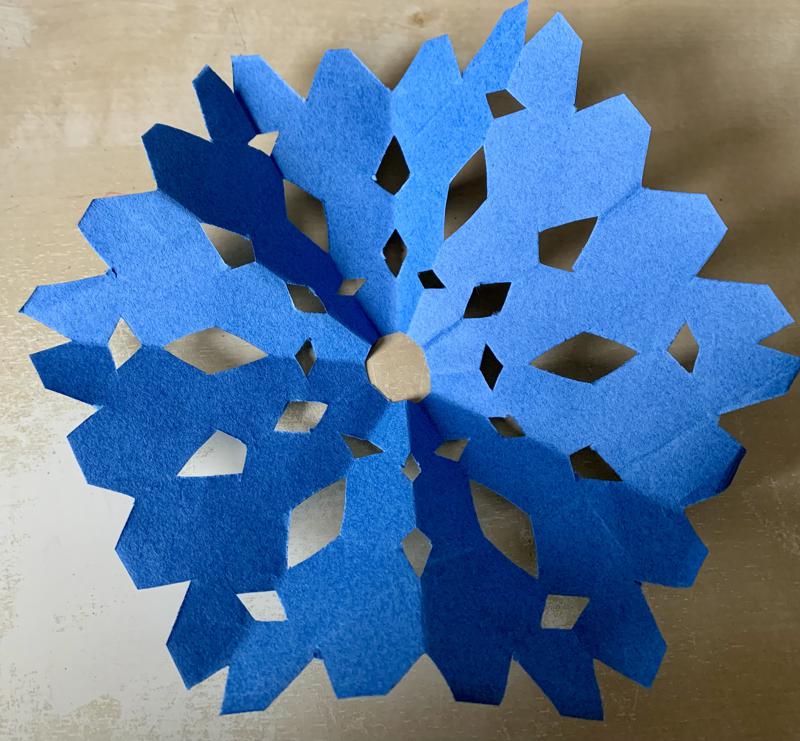
Remember back in elementary school, where you made paper snowflakes by folding a square piece of paper into a small triangle and then cut notches into it? One thing that always bothered me is that you end up with a square snowflake, whereas real snowflakes are hexagonal. Recently, when I was doing craft projects with my daughter, I wondered if I could come up with a way to make hexagonal snowflakes.
After some trial and error, here’s what I came up with. (I suspect that this construction is already known, e.g., by people who have studied origami.)
In these diagrams, green lines represent the folds to make in that step, white lines represent pre-existing creases, and purple lines represent the cuts to make in that step.
First, fold a piece of paper in half lengthwise.
Next, fold the paper in half lengthwise, again.
Unfold the paper. The paper should now be divided into four equal strips lengthwise. Fold the paper in half widthwise.
Next, fold the paper in half again, along the first crease you made. Orient the resulting twice-folded paper so that the folded edges are on the top and right, and the paper's original edge are on the bottom and left.
Now, fold over the top left corner, so that the resulting crease starts at the top-right corner, and so the corner of the resulting fold touches the middle crease.

Flip the paper around and repeat the same move. Then unfold once, so that you have a half-sheet with two corners down, as in the diagram. Note that it's important that the two creases meet in the middle.

Now, make two new folds. Each of these folds will go through the corner of the previous folds, and the new crease should be perpendicular to the diagonal folded edge. Fold these flaps back. You want the top edge of the flaps on the back to line up with the top diagonal folded edge of the rest of the paper.


Now, make a crease between the two bottom corners of the flaps (parallel to the bottom edge of the paper and perpendicular to the middle crease). Then cut along that crease.
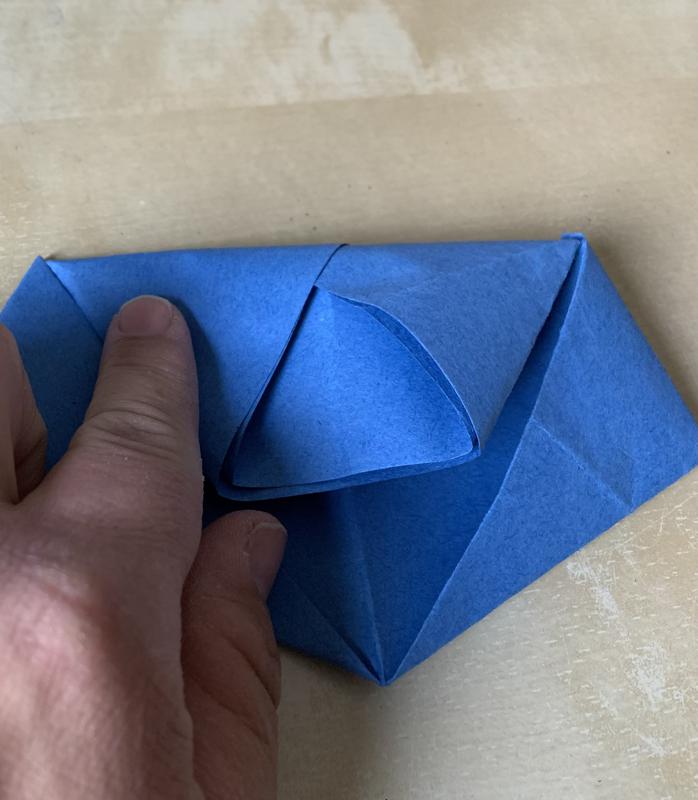
Next cut along the creases formed by folding the two flaps back.
Unfold the two flaps. At this point, you should have the bottom half of a hexagon. (If you unfolded completely, you would have a regular hexagon.) Fold the resulting shape into an equilateral triangle, by making new folds from each bottom corner to the top of the middle crease.
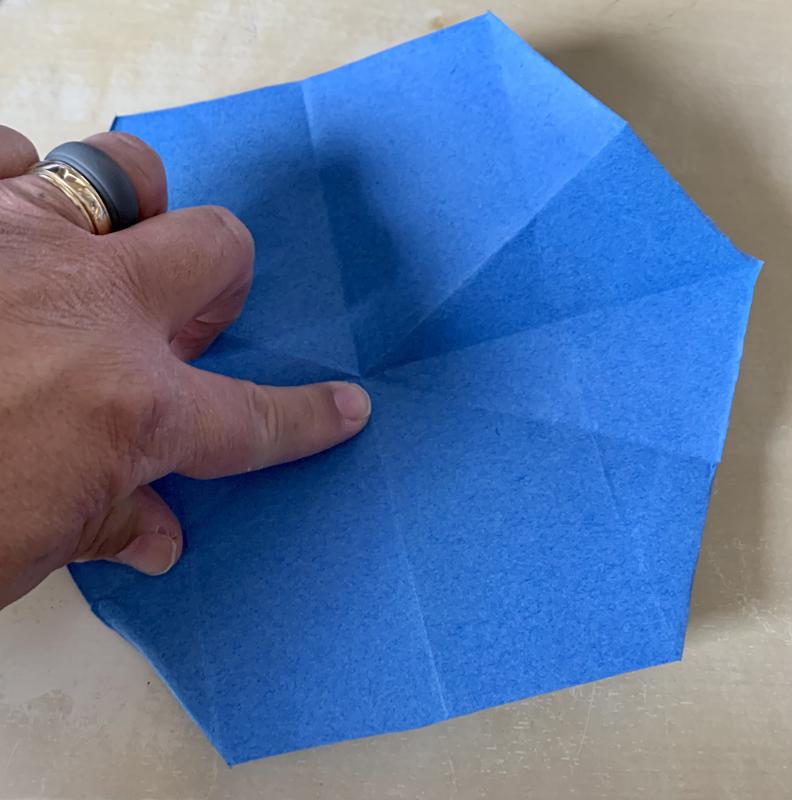
Fold the resulting triangle in half along the middle crease to make a right triangle. Cut out notches from the sides and corners as desired.
Unfold and you will have a hexagonal snowflake!
